Standard deviation
Standard deviation definition:
The square root of the average of the squares of the deviations of the respective data from their arithmetic mean is standard deviation definition.
It is a statistical measure that gives us an insight into the deviations of the terms from their arithmetic mean or from each other.
Standard deviation measures whether the terms are dense or scattered.
Usually average of the terms is taken as the standard point w.r.t. which the deviations of the terms are measured and consequently the standard deviation of the terms is found.
Use of standard deviation definition
Standard deviation definition is just sufficient to compare whether data in two different sets are spread out or dense without having to calculate the actual value of standard deviation using the formula for it.
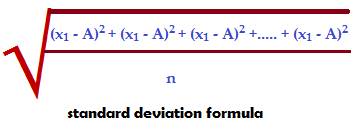
The Standard deviation formula
Consider ‘n’ terms denoted as x1, x2, x3, ... xn
Let A denote the average of the n terms.
Then, Standard deviation definition or formula of the n terms is
Example
Find the standard deviation of 2, 4, 6, 8, and 10
Solution:
The given terms are in arithmetic sequence,
Therefore, Average of the terms is same as median, the middle term, which is 6.
Find the sum of the squares of the deviations of each term from the mean 6 as below
(2 – 6)2 + (4 – 6)2 + (6 – 6)2 + (8 – 6)2 + (10 – 6)2 =
(–4)2 + (–2)2 + (0)2 + (2)2 + (4)2 =
16 + 4 + 0 + 4 + 16 = 40
After applying 40 in the standard deviation definition, we get
The numerical value 2√2 for standard deviation signifies a measure of deviations of the terms from their arithmetic mean.