Math-for-all-Grades.com
Time and Work
Lesson: Time and Work
In ratio and proportion, we learnt that there are two types of proportions:
Direct proportion and Indirect proportion.
If four numbers a, b, c and d are in direct proportion, then we write it as
a: b :: c: d

and from the rule:
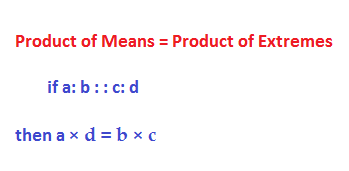
Example:
If 4 pencils cost $10, then what will 6 pencils cost?
Solution:
Number of pencils and their Cost are quantities that are directly proportional.
So, we can frame the expression
“ 4 is to 10 is same as 6 is to What Price P?
i.e. 4: 10 : : 6: P, then
4 × P = 10 × 6, so P = (10 × 6)/4 = 15
So, 6 pencils will cost $15
Now, consider the following example:
2 men complete a work in 1 day, then 1 man alone will finish the same work in how many days?
Solution:
Between Number of men and Amount of work, there is not a direct variation, rather there is an indirect proportion.
To finish a same amount of work, More men will take Less time, while Fewer men take More time
Now, from the Ratio and Proportion lesson, we know that if four quantities a, b, c and d are in indirect proportion, then we can set the relation as:
“ a varies to b indirectly as c varies to d” denoted as below:
a: b : : d: c, and
from the rule “ product of means is equal to product of extremes”, we write:
a × c = b × d
Now, if 2 men take 1 day, then 1 man will take what time can be set up as below:
2: 1 : : N: 1, so that we get
2 × 1 = N × 1, so N = 2 days.
Example 2:
If 3 men finish a work in 4 hours, then 4 men will finish the same work in what time?
Solution:
Since, Number of men and Time taken to finish the work are inversely proportional, we can write:
3 is to 4 is same as N is to 4, where N is the required number of days.
3: 4 : : N: 4.
From the rule, we write:
3 × 4 = 4 × N,
So, N = 3 days.
1. A Very Important Formula:
1. To finish a same amount of work, if M1 men take D1 days and M2 men take D2 days, then
M1 × D1 = M2 × D2
Let us now apply this formula to the above question:
M1 = 3, D1 = 4, M2 = 4 D2 =?
3 × 4 = 4 × D2
So, D2 = 3
2. Again, to finish the same amount of work, if M1 men take D1 days working H1 hours a day, and M2 men take D2 days working H2 hours a day, then we can write:
M1 × D1 × H1 = M2 × D2 × H2
Example:
To finish a same amount of work, if 4 men take 6 days working 5 hours a day, then how many days will 6 men take working 4 hours a day?
Solution:
Using the formula:
M1 × D1 × H1 = M2 × D2 × H2
4 × 6 × 5 = 6 × D2 × 4
We get: D2 = 5 days.
2. Another Very Important Concept:
One-day Work Concept:
To finish a same amount of work, a man takes 4 days while a woman takes 6 days, each working alone. If the two persons work together, in what time will they complete the work?
Solution:
Man takes 4 days,
Woman takes 6 days,
So, working together, both take 4 + 6, i.e. 10 days.
This is OBVIOUSLY WRONG.
Why?
Of course, two persons joining hands to finish off a work will naturally take less time than that taken by each one of them.
Here again, the concept of indirect proportion comes into play on Number of men and Time taken to complete a same amount of work.
Then, what is the way?
Here goes the solution:
If the man completes the work in 4 days, then in each one of the 4 days, he will complete 1/4 th of the total work.
(It is legitimately assumed in Time and Work questions that work done in each day is a same part of the whole)
So, the Man will complete a ¼ th on the first day, another ¼ th on the 2nd day, a third 1/4th on the 3rd day, and the final ¼ th on the 4th day:
So, the total amount of work (denoted by number “1”) on each of the 4 days is:
¼ + ¼ + ¼ + ¼ = 1
So, there we get to see one very important concept in Time and Work:
If a person completes alone a work in N days, then in one day the part of the work done is 1/N
Note:
“Be careful to note that the fraction for one-day work is a reciprocal of the number for time taken to finish the whole work
Plainly speaking, one-day work and number of days are numbers reciprocals of each other”
(Fraction ¼ for one-day work is reciprocal of 4, the number for time taken to finish the whole work)
This is the concept of One-Day Work:
Now, applying this one-day work concept to the above example:
Work completed in One day by the
Man = 1/4,
Woman = 1/6,
Man & Woman together = 1/4 + 1/6 = 5/12
Therefore, number of days both take to complete the whole work is 12/5
Another Very Important Formula:
If one person A takes “x” days to complete a work alone and another person B takes “y” days to complete the same work alone, then the number days both A and B take working together is :
x y/(x + y)
Applying this formula to the above question, we see the man and woman will together take:
4 × 6/ (4 + 6) = 24/10 = 12/5 days.
A Very Important Formula:
If three persons A, B and C take “x”, “y” and “z” days respectively to complete a work working alone, then the number of days taken by all three working together is:
x y z/(xy + yz + xz)
Example:
Working at their own individual rates, three persons finish a same work in 4, 5 and 6 days respectively. In what time will they complete the work together?
Solution:
Apply the above formula:
(4 × 5 × 6) / (4 × 5 + 5 × 6 + 4 × 6) = 120/74 =60/37 days.




